A mathematical constant is a key number whose value is fixed by an unambiguous definition, often referred to by a special symbol (e.g., an alphabet letter), or by mathematicians' names to facilitate using it across multiple mathematical problems.^[1] Constants arise in many areas of mathematics, with constants such as e and π occurring in such diverse contexts as geometry, number theory, statistics, and calculus.

Some constants arise naturally by a fundamental principle or intrinsic property, such as the ratio between the circumference and diameter of a circle (π). Other constants are notable more for historical reasons than for their mathematical properties. The more popular constants have been studied throughout the ages and computed to many decimal places.

All named mathematical constants are definable numbers, and usually are also computable numbers (Chaitin's constant being a significant exception).

Basic mathematical constants

These are constants which one is likely to encounter during pre-college education in many countries.

Archimedes' constant π

The constant π (pi) has a natural definition in Euclidean geometry as the ratio between the circumference and diameter of a circle. It may be found in many other places in mathematics: for example, the Gaussian integral, the complex roots of unity, and Cauchy distributions in probability. However, its ubiquity is not limited to pure mathematics. It appears in many formulas in physics, and several physical constants are most naturally defined with π or its reciprocal factored out. For example, the ground state wave function of the hydrogen atom is

${\displaystyle \psi (\mathbf {r} )={\frac {1}{\sqrt {\pi {a_{0}}^{3}}}}e^{-r/a_{0}},}$

where ${\displaystyle a_{0}}$ is the Bohr radius.

π is an irrational number and a transcendental number.

The numeric value of π is approximately 3.1415926536 (sequence A000796 in the OEIS). Memorizing increasingly precise digits of π is a world record pursuit.

The imaginary unit i

The imaginary unit or unit imaginary number, denoted as i, is a mathematical concept which extends the real number system ${\displaystyle \mathbb {R} }$ to the complex number system ${\displaystyle \mathbb {C} .}$ The imaginary unit's core property is that i² = −1. The term "imaginary" was coined because there is no (real) number having a negative square.

There are in fact two complex square roots of −1, namely i and −i, just as there are two complex square roots of every other real number (except zero, which has one double square root).

In contexts where the symbol i is ambiguous or problematic, j or the Greek iota (ι) is sometimes used. This is in particular the case in electrical engineering and control systems engineering, where the imaginary unit is often denoted by j, because i is commonly used to denote electric current.

Euler's number e

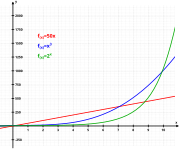
Euler's number e, also known as the exponential growth constant, appears in many areas of mathematics, and one possible definition of it is the value of the following expression:

${\displaystyle e=\lim _{n\to \infty }\left(1+{\frac {1}{n}}\right)^{n}}$

The constant e is intrinsically related to the exponential function ${\displaystyle x\mapsto e^{x}}$.

The Swiss mathematician Jacob Bernoulli discovered that e arises in compound interest: If an account starts at $1, and yields interest at annual rate R, then as the number of compounding periods per year tends to infinity (a situation known as continuous compounding), the amount of money at the end of the year will approach e^R dollars.

The constant e also has applications to probability theory, where it arises in a way not obviously related to exponential growth. As an example, suppose that a slot machine with a one in n probability of winning is played n times, then for large n (e.g., one million), the probability that nothing will be won will tend to 1/e as n tends to infinity.

Another application of e, discovered in part by Jacob Bernoulli along with French mathematician Pierre Raymond de Montmort, is in the problem of derangements, also known as the hat check problem.^[2] Here, n guests are invited to a party, and at the door each guest checks his hat with the butler, who then places them into labelled boxes. The butler does not know the name of the guests, and hence must put them into boxes selected at random. The problem of de Montmort is: what is the probability that none of the hats gets put into the right box. The answer is

${\displaystyle p_{n}=1-{\frac {1}{1!}}+{\frac {1}{2!}}-{\frac {1}{3!}}+\cdots +(-1)^{n}{\frac {1}{n!}}}$

which, as n tends to infinity, approaches 1/e.

e is an irrational number and a transcendental number.

The numeric value of e is approximately 2.7182818284 (sequence A001113 in the OEIS).

Pythagoras' constant √2

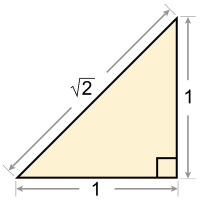
The square root of 2, often known as root 2, radical 2, or Pythagoras' constant, and written as √2, is the positive algebraic number that, when multiplied by itself, gives the number 2. It is more precisely called the principal square root of 2, to distinguish it from the negative number with the same property.

Geometrically the square root of 2 is the length of a diagonal across a square with sides of one unit of length; this follows from the Pythagorean theorem. It was probably the first number known to be irrational. Its numerical value truncated to 65 decimal places is:

1.41421356237309504880168872420969807856967187537694807317667973799... (sequence A002193 in the OEIS).

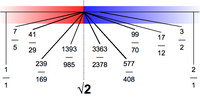
Alternatively, the quick approximation 99/70 (≈ 1.41429) for the square root of two was frequently used before the common use of electronic calculators and computers. Despite having a denominator of only 70, it differs from the correct value by less than 1/10,000 (approx. 7.2 × 10 ⁻⁵).

Theodorus' constant √3

The numeric value of √3 is approximately 1.7320508075 (sequence A002194 in the OEIS).

Constants in advanced mathematics

These are constants which are encountered frequently in higher mathematics.

The Feigenbaum constants α and δ

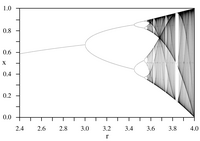
Iterations of continuous maps serve as the simplest examples of models for dynamical systems.^[3] Named after mathematical physicist Mitchell Feigenbaum, the two Feigenbaum constants appear in such iterative processes: they are mathematical invariants of logistic maps with quadratic maximum points^[4] and their bifurcation diagrams. Specifically, the constant α is the ratio between the width of a tine and the width of one of its two subtines, and the constant δ is the limiting ratio of each bifurcation interval to the next between every period-doubling bifurcation.

The logistic map is a polynomial mapping, often cited as an archetypal example of how chaotic behaviour can arise from very simple non-linear dynamical equations. The map was popularized in a seminal 1976 paper by the Australian biologist Robert May,^[5] in part as a discrete-time demographic model analogous to the logistic equation first created by Pierre François Verhulst. The difference equation is intended to capture the two effects of reproduction and starvation.

The numeric value of α is approximately 2.5029. The numeric value of δ is approximately 4.6692.

Apéry's constant ζ(3)

Apery's constant is the sum of the series

$${\displaystyle \zeta (3)=1+{\frac {1}{2^{3}}}+{\frac {1}{3^{3}}}+{\frac {1}{4^{3}}}+\cdots }$$

Despite being a special value of the Riemann zeta function, Apéry's constant arises naturally in a number of physical problems, including in the second- and third-order terms of the electron's gyromagnetic ratio, computed using quantum electrodynamics.^[6]

The golden ratio φ

${\displaystyle F_{n}={\frac {\varphi ^{n}-(1-\varphi )^{n}}{\sqrt {5}}}}$

An explicit formula for the nth Fibonacci number involving the golden ratio φ.

The number φ, also called the golden ratio, turns up frequently in geometry, particularly in figures with pentagonal symmetry. Indeed, the length of a regular pentagon's diagonal is φ times its side. The vertices of a regular icosahedron are those of three mutually orthogonal golden rectangles. Also, it appears in the Fibonacci sequence, related to growth by recursion.^[7] Kepler proved that it is the limit of the ratio of consecutive Fibonacci numbers.^[8] The golden ratio has the slowest convergence of any irrational number.^[9] It is, for that reason, one of the worst cases of Lagrange's approximation theorem and it is an extremal case of the Hurwitz inequality for Diophantine approximations. This may be why angles close to the golden ratio often show up in phyllotaxis (the growth of plants).^[10] It is approximately equal to 1.6180339887498948482, or, more precisely 2⋅sin(54°) = ${\displaystyle \scriptstyle {\frac {1+{\sqrt {5}}}{2}}.}$

The Euler–Mascheroni constant γ

The Euler–Mascheroni constant is defined as the following limit:

${\displaystyle {\begin{aligned}\gamma &=\lim _{n\to \infty }\left(\left(\sum _{k=1}^{n}{\frac {1}{k}}\right)-\ln n\right)\\\end{aligned}}}$

The Euler–Mascheroni constant appears in Mertens' third theorem and has relations to the gamma function, the zeta function and many different integrals and series.

It is yet unknown whether ${\displaystyle \gamma }$ is rational or not.

The numeric value of ${\displaystyle \gamma }$ is approximately 0.57721.

Conway's constant λ